Stable Diffusion Model
Table of Contents
What is Stable Diffusion Model?
The Stable Diffusion Model is a mathematical model that is used to describe the spread of information, ideas, or innovations through a population over time. This model is based on the concept of diffusion, which is the process by which something spreads or moves from one place to another.
The Stable Diffusion Model is often used in the field of social sciences to study how new ideas or innovations are adopted by individuals within a society. It can also be applied to other areas, such as marketing, economics, and epidemiology, to understand how trends or behaviors spread through a population.
Understanding the Stable Diffusion Model
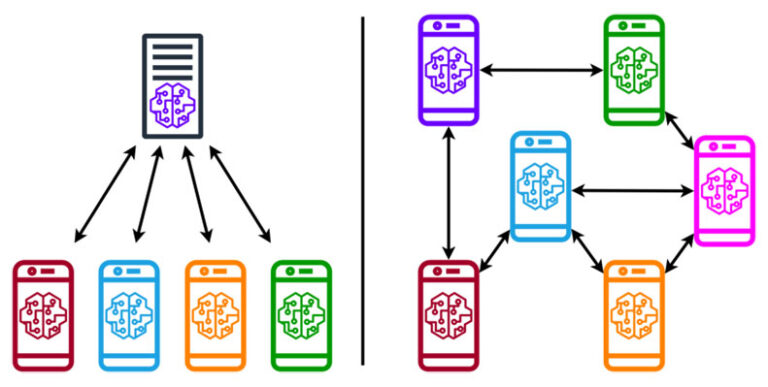
The Stable Diffusion Model is based on the idea that the rate at which something spreads through a population is influenced by several factors, including the characteristics of the individuals within the population, the nature of the thing being spread, and the social networks through which the spread occurs.
One of the key components of the Stable Diffusion Model is the concept of stability, which refers to the tendency of a trend or behavior to persist over time. In other words, a trend that is stable is more likely to continue spreading through a population than one that is unstable.
Another important aspect of the Stable Diffusion Model is the idea of diffusion speed, which is the rate at which something spreads through a population. This speed can vary depending on factors such as the size of the population, the density of social networks, and the presence of barriers to diffusion.
Applications
The Stable Diffusion Model has been used in a variety of fields to study the spread of trends, behaviors, and innovations. In the field of marketing, for example, the model can be used to understand how new products or services are adopted by consumers and to develop strategies for marketing these products effectively.
In economics, the Stable Diffusion Model can be used to study how new technologies or business practices are adopted by firms and to analyze the impact of these adoptions on the economy as a whole. The model can also be applied to epidemiology to study the spread of diseases through a population and to develop strategies for controlling or preventing outbreaks.
The Stable Diffusion Model in Action
To illustrate how the Stable Diffusion Model works in practice, let’s consider an example of a new technology being adopted by a population. Suppose a company has developed a new smartphone that offers advanced features and capabilities.
At first, only a small number of individuals within the population are aware of the new smartphone and its benefits. These early adopters may begin using the smartphone and spreading the word to their friends and family members.
As more people hear about the new smartphone and see its benefits in action, they may become interested in adopting the technology themselves. This creates a ripple effect, with more and more individuals within the population adopting the smartphone over time.
As the smartphone becomes more popular and widely adopted, the rate of diffusion increases, and the technology may eventually reach a point of saturation, where almost everyone within the population has adopted the smartphone.
By studying the adoption of the new smartphone using the Stable Diffusion Model, researchers can gain insights into the factors that influence the spread of innovations through a population and develop strategies for promoting the adoption of new technologies in the future.
Conclusion
In conclusion, the Stable Diffusion Model is a powerful tool for studying how trends, behaviors, and innovations spread through a population over time.
By understanding the factors that influence diffusion speed and stability, researchers can gain valuable insights into the dynamics of social networks and develop strategies for promoting the adoption of new ideas or technologies.
Whether applied to marketing, economics, or epidemiology, the Stable Diffusion Model offers a valuable framework for understanding the complexities of diffusion processes in a variety of contexts.